Why Teaching Properties of Real Numbers is Important

What Good Is It?

The Real Number Line has always been one of the dullest lessons I have to teach.

 Natural Numbers are the set of numbers you can count on your fingers, beginning with one.  The Whole Numbers are the Natural Numbers and Zero...Integers are ...

Blah Blah Blah

I have to teach it because it's in the curriculum.  And I always wonder, what use is it if a student knows the difference between a whole number and a natural number?

It is hypocritical of me to complain in such a fashion because I laud the virtues of education being greater than a set of skills or a body of knowledge.  Education is about learning to think, uncovering something previously unknown that ignites excitement and interest.  Education should change how you see yourself, how you think about the world.  It should enrich our lives.

Teaching the Real Number Line can be a huge first step in that direction, if done properly.

Math is About Ideas, Not Just Computation

There are some rich, yet entirely approachable, mathematical ideas that can be introduced with the Real Number Line (RNL).  For example, a series of questions to be posed to students could be:

1.  The Natural Numbers are infinite, meaning, they cannot be counted entirely.  How do we know that?
2. The Integers are also infinite.  How do we know that?
3.  Is infinity a number?
4. Which are there more of, Natural Numbers of Integers?  How can you know, if they're both infinite?

The idea of an axiom can be introduced.  Most likely, students assume math is true, or entirely made up, but correct or incorrect, because it is written in a book and claimed to be such by a teacher.  The idea of how we know what we know and if math is an invention or a discovery can be introduced by talking about axioms.  For example:

1. Is it true that 5 + 4 = 4 + 5 ?
2. If a and b are Real Numbers, would it always be true that a + b = a + b?  (What if they were negative?)
3. Is it also true that a - b = b - a?  How do we know that?
4. Is the following also true:  If a = b, and b = c, then a = c?  How do we know?

The idea here is not to teach students the difference between the Associative Property and the Commutative Property, but to use these properties to introduce students to math as a topic that can be discussed, and that it is not about answer getting, but instead about ideas.

For more on this topic and a few other related items, visit this page.

Why Are Some Rational Numbers Non-Terminating Decimals?

If you had a particularly smart group of students, you could pose this question.  I mean, after all, 1/3 = 0.3333333333333333...  And yet, we are told rational numbers include decimals that can be written as a fraction (the ratio of two integers).

How it works is sometimes very clear and clean.  For example, 0.7 is said, "Seven tenths." And "Seven tenths," can also be written as the ratio of seven and ten.  And the number seven tenths is of course equal to itself, regardless of how it is written.  The number 0.27 is said, "twenty seven hundredths," which can easily be written as the ratio of twenty seven, for the numerator, and one hundred, as the denominator.  And this can continue so long as the decimal terminates.  But try the same thing with the a repeating decimal and you do not end up with things that are equal.

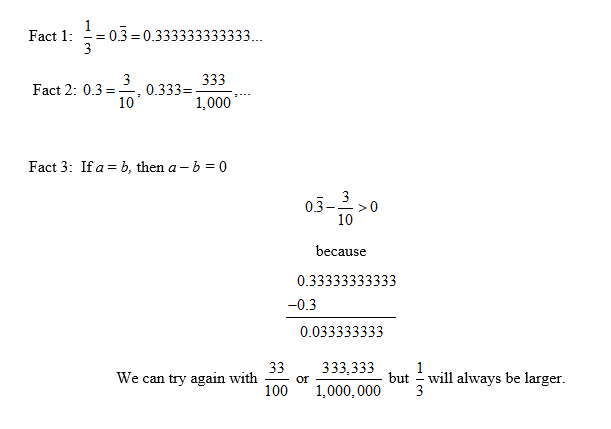
The algorithm to convert a repeating, but non-terminator decimal into a fraction is pretty straight forward.

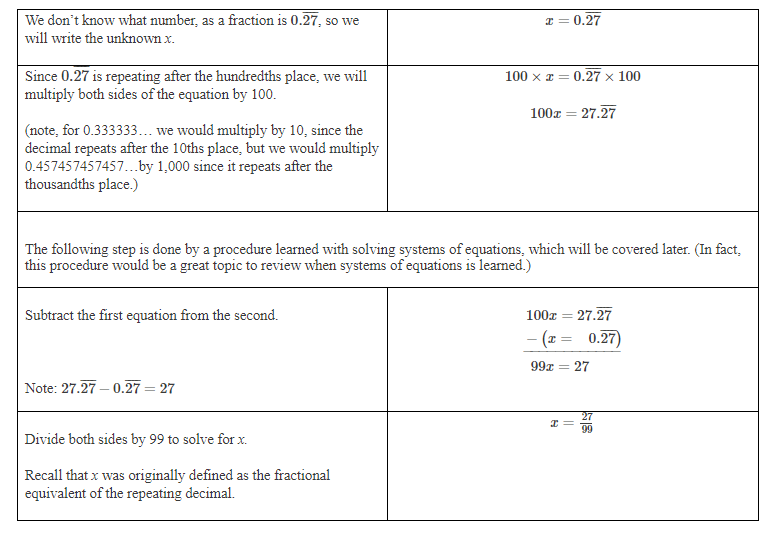
But that does not address why a rational number would be a non-terminating decimal.

Click the PPT Icon to the left to download a lesson on converting repeating decimals into fractions for honors students.  It includes a proof of why the square root of two is irrational.

The simple reason that some rational numbers cannot be expressed as terminating decimals has to do with our numbering system.  We use base 10 numbers.  Our decimal system provides us an easy way to write fractions with denominators that are powers of 10.

That means that we have ten numbers, including zero, that fill up one column, like a car’s odometer.  When you travel 9 miles the odometer will read 000009.  When you travel the tenth mile the odometer will read 000010.

Not all of our number systems are base ten. While the metric system is base 10, or at least translates into powers of ten, the Imperial system is base 12 from inches to feet, but 5,280 feet to the next unit of miles, and so on.

Time is another great example of bases other than ten.  Seconds and minutes are base sixty.  You need sixty seconds before you have an hour, not ten.  But hours are base 24 because 24 hours are needed to make one of the next category, which is days.

In time, 25 minutes of an hour is the ratio: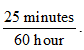 

But in base ten this is 0.4166666666666666... Our decimal system does math in base ten, not base sixty.  This is not 41 minutes!  A typical mistake would be two say 25 minutes is 0.25 of an hour.

Back to our original example of 1/3.  Not all numbers can be cleanly divided into groups of ten, like 3.  If we had a base 3 numbering system, where after the third number we moved, then 1/3 would just be 0.1.  But in our numbering system, 0.1 is one tenth.

Other numbers, like four, translate into ten more easily.  Consider the following:

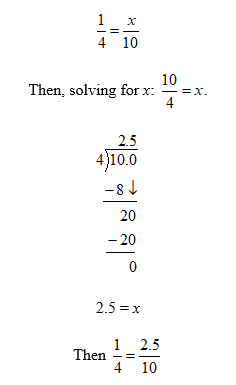
The only issue remaining is that 2.5/10 is not a rational number because 2.5 is not an integer and rational numbers are ratios of two integers.  This can be resolved as follows:

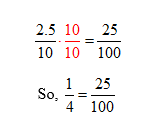
Let's try the same process with 1/3.

As you can see, we will keep getting ten divided by three, forever.

This is a great example of how exploring a question can uncover many topics within the scope of the course being taught.

I hope this has caused you to pause and think of how exploring questions, relationships and properties in mathematics can lead to greater understanding than just teaching process and answer getting.

Here is a PowerPoint presentation you can download and use in your class.