Percentages, Interest, Profit and Growth
Part 1: Review
This is perhaps one of the best topics to help promote the ability to write and read algebraic equations. If you’re a student, reading this, do not skip writing the equations, even when the problems seem easy. Skipping this important step robs you of an opportunity to learn and practice a skill that will be required later. And if you’re trying to learn it later it will be incredibly difficult. If you’re a teacher reading this, do not become too predictable in your style of questioning. Do not allow students to fall into a groove where if a problem looks like this, they just do that and they’re done. In other words, it is your job to now allow students to figure you out and think they’ve learned the math.
Before we begin with the new materials that relates to percentages, let us lay some ground rules, a quick review. Even if you already know the following, a little refresher will not hurt.
A ratio compares two numbers. They’re very important because they allow us to communicate important relationships. For example, I believe there is an important ratio of peanut butter to jelly on a sandwich. I prefer approximately 3:1 peanut butter to jelly.
Ratios written like 3:1 do not lend themselves to mathematical manipulation, but when written as a fraction, much more robust mathematical manipulation is available. Percentages are ratios, usually written as a number with a percent symbol (%). But that percent symbol means a number divided by 100. Percentages are ratios with a fixed denominator.
Regardless of procedure, when changing a number into a percentage, what is really happening is we are writing a number as a ratio where the denominator is 100. All of the following fit this:
Change the following into a percentage:
a) 1.54
b) 23
c) 67/90
d)
Example a) is pretty simple because decimals are fraction with denominators that are powers of 10. The second decimals place is 100, which is what we want. This is 154/100! It is 154%. The % symbol means divided by 100.
The second example, b), is not so straight forward. Students would be tempted to say it is 23%, but that would be 0.23 as a decimal, which is far different than 23. Twenty-three, as a percentage is really calculated as follows:
In the equation above I used a question mark instead of a variable only to get your attention to make this one small distinction. There is a difference between a variable and an unknown. A variable is a value that has many possible values that maintain truth (keep the equation true). An unknown, like we have here, has only one solution (value that maintains truth).
This equation is also a proportion. A proportion is two ratios that are equal. To make 23 a percentage, we must rewrite it as a fraction with a denominator of 100. We do not know what the numerator is going to be, but we can change 1 into 100 by multiplying.
Which gives us:
A percentage is a ratio of a number and one hundred. So, 23 is 2,300%.
Example c), is slightly tricker because changing 90 into 100 is not as clean as changing 1 into a 100 (with multiplication).
Let’s do a quick check for understanding. Which of the following is true, and why is the other false.
To change 67/90 into a percentage we need the following figure out the following:
The problem is that there is no way to make 90 into 100 by integer multiplication. The next best way to perform this translation is to rewrite 67/90 as a decimal and round to the 100ths place by dividing 67 by 90.
This number, 67/90 cannot be accurately written as a percentage, we must approximate and round.
As a side note: The easiest way to convert a fraction into a percentage is to change the denominator into 100. But that only is possible if the factors of the denominator only contain prime factors of 2 and 5. (See why some rational numbers do not terminate.)
Example d) is an example of where we can change the denominator (4) into 100 with multiplication easily. We just need to multiply by 25, giving us = 25%.
Writing Equations with Percentages
Part 2
While shopping at your local international market you come across a discounted jar of marmite. You’re curious who would eat such a thing and decide to buy it. It is only sale, 15% off, with a discounted price of $4.50. How does a store arrive at such a price of $4.50 when something has been discounted by 15%? Are they just making things up and nobody questions them?
Let’s look carefully at how this calculation is carried out because we will be tackling tricky questions that rely on this level of understanding.
The difficult and confusing portion here revolves around the discounted price. We do not actually subtract a percentage from the original price but instead subtract a percentage of the original price from the original price. The discounted amount is that percentage of the original. This might seem confusing, but it will come into play very soon.
So how do we find the discounted amount? I offer two possible methods.
Method 1:
Original Price Original Price × Percentage Saved = Sale Price
This is the most familiar method and one which I believe retailers prefer us to think about. However, the second method is perhaps what should be considered because when we purchase something we are not saving money at all. The second method considers how much of the original is being spent.
Method 2:
Original Price × (100 Percentage Saved) = Sale Price
The other issue difference between the two methods is that Method 1 will provide more opportunities for calculation error because you will be dealing with larger numbers.
Let’s see how this works.
Example: The original price of bologna was $4.95 for 12 oz. Since it is now green and moldy it is on sale, reduced by 80%. What should the price be now?
Method 1:
Original Price Original Price × Percentage Saved = Sale Price
Method 2:
Original Price × (100 Percentage Saved) = Sale Price
I prefer the second method because the calculation of the percentage can be done in your head, leaving only one potentially tricky calculation. The first method has two tricky calculations.
Now back to the marmite. How much was it originally? The thing you must recognize is that where you place numbers, in either method’s formula, matters. To discover the original price of the marmite we must recognize that we must solve an equation. These formulas are not set up to find the original price, or percentage saved, by simple calculation. A simple inverse operation is required.
For both of these methods, let x be the original price.
Method 1:
Original Price Original Price × Percentage Saved = Sale Price
Since this is the beginning of an introductory Algebra course, you may not possess the skills to solve that equation, which would require factoring. However, method 2 is much simpler.
Method 2:
Original Price × (100 Percentage Saved) = Sale Price
Do you see now that this is the question, Eighty-five percent of what is 4.5?
x = 5.29
Let’s take this one step further. Suppose there was a zombie outbreak, and on July 1st, there were 200 zombies in your town. On July 2nd it increased by 25%. If we wanted to know how many zombies there were on July 2nd, we could find out one of two methods.
Method 1:
Original + Original × Percentage Growth = New Amount
Method 2:
Original × (100 + Percentage Growth) = New Amount
This might look similar, but in practice it is entirely different.
If something grows by 25%, wouldn’t there be 125% of the original? That would be 100% of the original amount plus the growth of 25%. That’s how Method 2 approaches these situations. Similarly, if there was a 25% reduction, would you have 75% of the original?
Profit, Growth and Percentages
Profit is the difference of the investment and the income. Investment is the amount of money used to begin the business (merchandise purchased, labor …). Income is the amount of money received in return for the efforts.
To find the percentage of profit we can use the following formula:
That is surely simple enough. So long as you refer to this relationship, you will not get too confused. The issue students experience with this entire section, is once again, they begin calculation before writing equations.
Since profit is investment income, we can further define percent profit as:
Perhaps you recognize this as percentage of growth. Percent profit and percentage growth are the same thing, the only difference being jargon.
The following two questions are the same category of question.
1.
The population of rabid dogs in Arizona grew
from 250 to 5,000 in three years. What
is the percentage of growth in that three year period?
2. You invest $5000 in a rabid dog removal company. Over a three year period you receive $12,500 in income. What’s your percentage of profit?
I will not write out how to answer these questions here because that is not help students avoid the pitfalls presented here. Instead, I will offer the following insight. In each problem, carefully identify what each number represents. Is the number a final value, original value or percentage of growth (or profit)?
Once those are determined it is as simple as replacing values and performing the calculations.
Let us look at one more problem along these lines. Later in this course we will be solving the equation we will write for this question, but for now we will just write the equation.
3. A house is sold for $125,000, which was a 5% profit. Write an equation that could be solved to find the original cost of the home?
To answer this question we must identify that we are provided with percentage profit (or growth) and the final value. We are being asked to find out how much the house cost when the current sellers bought (or built) it.
We will be using the following equation:
The percentage of profit is 5%. The income value is $125,000. The original cost will be the investment.
This is a tricky equation to solve, it would involve factoring, which will be learned at a later point. But for now, writing the equations involved is of utmost importance.
Interest
Part 3
Interest is also growth, but defined as a percentage of an initial amount (investment or principal) over time. There are two types of interest discussed here, simple and compound. There is a third type, continuously compound interest, but that will be discussed later.
Interest is a percentage of the original amount (principal). Let us consider this statement and translate it into a mathematical equation. Let’s us I for interest, r for the percent (rate) and P for principal.
Interest is a percent of the original amount (principal)
I = r × P
Normally we see this written as: I = Pr, which is the same thing since when letters are written next to each other in math the inferred operation is multiplication and the order in which you multiply does not matter (Pr = rP).
Interest is added to the principal (original) amount after it is calculated. So if you owe someone money, as in a loan, and there is interest charged, you will owe the amount you borrowed plus the interest. The amount owed (A for amount) is the principal (P for principal) plus the interest (I).
That gives us the following equation:
A = P + I
Let us first consider Simple Interest. Simple Interest adds the interest to the amount every time period (we will use years most of the time). For example, if you earn 50% interest on $100 annually, you’ll earn $50 every year.
Consider the following table:
|
Year |
Interest |
Amount |
|
0 |
0 |
100 |
|
1 |
50 |
150 |
|
2 |
50 |
200 |
|
3 |
50 |
250 |
|
4 |
50 |
300 |
|
5 |
50 |
350 |
|
6 |
50 |
400 |
|
7 |
50 |
450 |
|
8 |
50 |
500 |
|
9 |
50 |
550 |
|
10 |
50 |
600 |
Notice the amount increases annually because the interest (I = Pr) is added to it every time period. To calculate this we could add the interest repeatedly, for however many time periods were given.
A = P + Pr + Pr + Pr + Pr … + Pr
But since we are adding the same amount, repeatedly, we could multiply. Remember, multiplication is repeated addition of the same amount.
Using t for time, we can find the amount with the following equation:
A = P + Prt
That would give us the amount is the principal plus all of the interest.
The key difference between Simple Interest (as shown above) and Compound Interest (which follows) is that the amount of interest being added to the amount stays the same with Simple Interest. Every year we add the percent-interest of the principal to the total.
Compound interest is the same for only the first time period. Let’s see how we calculate compound interest.
Year 1:
Interest: Amount × Percent
$100×0.50 = $50
Amount: Current Amount + Interest Earned
$100 +$50 = $150
Make sure you notice there is a difference between the interest earned and the amount. Take care to read the questions you are asked carefully as you could be asked for one or the other and they are not the same thing.
Year 2:
Interest: Amount × Percent
$150 × 0.50 = $75
Amount: Current Amount + Interest Earned
$150 + $75 = $225
Year 3:
Interest: Amount × Percent
$225 × 0.50 = $112.50
Amount: Current Amount + Interest Earned
$225 + $112.50 = $337.50
As you can see, the amounts in compound interest grow much more quickly than with simple interest. This is because in compound interest the interest calculated each period is taken from the current amount, not the principal.
For three or fewer years, compound interest is easy enough to calculate in this fashion. But to fill out a table for 10 years, like we did with simple interest, this is cumbersome and the likelihood of a calculation error is enormous.
So, let’s see if we can make a formula. A simple search on the internet for compound interest provides us with this ugly formula:
This does work but provides no insight into the workings behind compound interest. Taking a step back, let us consider what happens when we calculate interest.
Interest is growth. Considering just one year, if you have 50% interest, you end up with 150% because you have 100% of what you started with, plus the 50% growth.
So instead of calculating amounts with interest as we did percentages earlier with Method 1:
Amount = Principal + Principal × Interest Rate,
We could consider this same calculation as with did earlier with Method 2:
Amount = Principal × (100% + Interest Rate)
Now if we consider the percentages as decimals, and just use the letter r for Interest Rate, P for principal and A for amount:
Amount = Principal × (1 + r)
The difference between compound interest and simple interest is compound interest adds each term’s interest earned back into the principal. So if the equation above was the first term (we will consider years for now), then the second year would be this:
Amount = [Previous Amount + Principal × (1 + r)]r
This gets very ugly, very quickly. We could clean this up with variables and notation, but the take-away is that every year we are multiplying by the rate. Repeated multiplication can be written with exponents.
Amount = Principal × (1 + r)t
Using our formula for the example before, we would get:
In the formula you will find in an internet search is the same thing, except it considers that you may be compounding the interest more frequently than once a year. In later math we will get into that and see where the number e comes from. But for what we will need now, calculate compound interest either manually if it is a short number of years and a calculator is not present, or with the formula below.
Amount with Compound Interest
Number Unit
1.4 Algebraic Fractions (Part 1)
1.4.1 Algebraic Fractions (Part 2)
1.5 Order of Operations & Function Notation
1.10 Units, Ratios and Rates
The last and final thing we need to see here is how compound and simple interest differ in application. Consider the table below.
| Year | Interest | Amount
(simple) |
Amount
(compound) |
| 0 | 0 | 100 | 100 |
| 1 | 50 | 150 | 150 |
| 2 | 50 | 200 | 225 |
| 3 | 50 | 250 | 337.50 |
| 4 | 50 | 300 | 506.25 |
| 5 | 50 | 350 | 759.38 |
| 6 | 50 | 400 | 1139.06 |
| 7 | 50 | 450 | 1708.59 |
| 8 | 50 | 500 | 2562.89 |
| 9 | 50 | 550 | 3844.34 |
| 10 | 50 | 600 | 5766.50 |
Simple interest grows very by comparison to compound interest because compound interest calculates interest of interest already calculated. So it grows exponentially.
Let us consider a graph of these two types of interest.
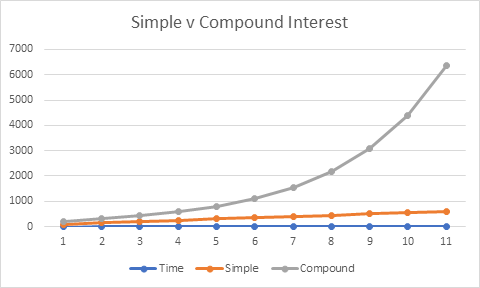
A graph, in one sense, is a visual representation of data. In this case, the amount of money as it grows with Simple versus Compound Interest. As we can see in the graph, the Compound Interest grows much faster than the Simple Interest. The simple interest is a line, which is called a linear relationship (linear comes from the word line, and all lines are straight). Compound interest is exponential, which means it is going to increase at an increasing rate.
These are key concepts for later in Algebra.
For practice problems, click here.



 For Teachers
For Teachers


